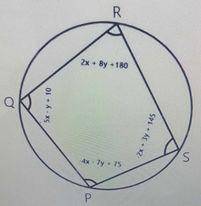
PQRS is a cyclic quadrilateral. Find x + y
50
60
15
0
Correct answer is D
∠PQR + ∠PSR = 180o (opp. angles of cyclic quad. are supplementary)
⇒ 5x - y + 10 + (-2x + 3y + 145) = 180
⇒ 5x - y + 10 - 2x + 3y + 145 = 180
⇒ 3x + 2y + 155 = 180
⇒ 3x + 2y = 180 - 155
⇒ 3x + 2y = 25 ----- (i)
∠QPS + ∠QRS = 180o (opp. angles of cyclic quad. are supplementary)
⇒ -4x - 7y + 150 + (2x + 8y + 105) = 180
⇒ -4x - 7y + 75 + 2x + 8y + 180 = 180
⇒ -2x + y + 255 = 180
⇒ -2x + y = 180 - 255
⇒ -2x + y = -75 ------- (ii)
⇒ y = -75 + 2x -------- (iii)
Substitute (-75 + 2x) for y in equation (i)
⇒ 3x + 2(-75 + 2x) = 25
⇒ 3x - 150 + 4x = 25
⇒ 7x = 25 + 150
⇒ 7x = 175
⇒ x=1757=25
From equation (iii)
⇒ y = -75 + 2(25) = -75 + 50
⇒ y = -25
∴ x + y = 25 + (-25) = 0
10 cm and 15 cm
8 cm and 12 cm
6 cm and 9 cm
12 cm and 18 cm
Correct answer is A
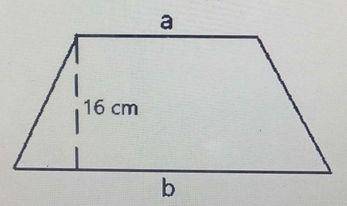
Area of trapezium = 12(a+b)h
⇒ 12(a+b)×16=200
⇒ 8(a + b) = 200
⇒ a + b = 2008 = 25 -----(i)
⇒ a : b = 2 : 3
⇒ ab=23
⇒ 3a = 2b
⇒ a = 2b3 -------(ii)
Substitute 2b3 for a in equation (i)
⇒ 2b3 + b = 25
5b3 = 25
⇒ b = 25 ÷ 53=25×35=15cm
From equation (ii)
⇒ a = 2×153=2×5=10cm
∴ Lengths of each parallel sides are 10cm and 15cm

Study the given histogram above and answer the question that follows.
What is the total number of students that scored at most 50 marks?
380
340
360
240
Correct answer is C
Total number of students that scored at most 50 marks = 100 + 80 + 60 + 40 + 80 = 360
Find the volume of a cone which has a base radius of 5 cm and slant height of 13 cm.
300π cm3
325π cm3
3253π cm3
100π cm3
Correct answer is D
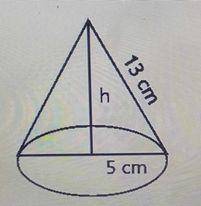
Volume of a cone = 13πr2h
r = 5 cm
l = 13 cm
Using Pythagoras theorem
⇒ 132=52+h2
⇒ 169=25+h2
⇒ 169−25=h2
⇒ h2=144
⇒ h=√144=12cm
∴ Volume of the cone = 13×π×52x12=100π cm3
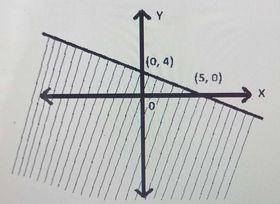
Which inequality describes the graph above?
4y+5x≥20
5y+4x≤20
4y+5x≤20
5y+4x≥20
Correct answer is B
First, we find the equation of the boundary line using the two intercepts.The slope is
m = 4−00−5=45
The y-intercept is 4
The slope-intercept form of the equation is therefore
y = -45x+4
⟹y+45x=4
Multiply both sides by 54
⟹54(y+45x)=4×54
⟹54y+x=5
Multiply both sides by 4
⇒ 5y+4x=20
The inequality is therefore either 5y+4x≤20 or 5y+4x≥20.
Using the test point (0, 0) -The origin
⇒ 5(0) + 4(0) ≤ 20
⇒ 0 ≤ 20 (True)
∴ The inequality is 5y+4x≤20
JAMB Subjects
Aptitude Tests