Mathematics Questions and Answers

Total cost price = ₦9,200.00 + ₦1,500.00 = ₦10,700.00

Selling price = ₦13,400.00

Gain = ₦13,400.00 - ₦10,700.00 = ₦2,700.00

∴ % gain = $\frac{₦2,700.00}{₦10,700.00}\times$ 100% = 25.23%

Using Pythagoras theorem

⇒ $(20\sqrt2)2 = x^2 + x^2$

⇒ 800 = $2x^2$

⇒ 400 = $x^2$

⇒ x = $\sqrt400$ = 20 cm

∴ Area of a square = $x^2 = 20^2 = 400 cm^2$

∴ Perimeter of a square = 4x = 4 x 20 = 80 cm

Volume of the composite solid = Volume of A + Volume of B

Volume of a cuboid = length x breadth x height

Volume of A = 6 x 26 x 8 = 1248 cm$^3$

Volume of B = 6 x 10 x 22 = 1320 cm$^3$

∴ Volume of the composite solid = 1248 + 1320 = 2568 cm$^3$

Total possible outcome = 6 x 6 = 36

Required outcome = 15

∴ Pr(E) = $\frac{15}{36} = \frac{5}{12}$

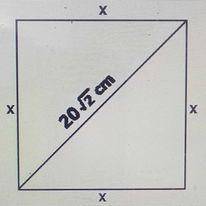
An exterior angle of a n-sided regular polygon = $\frac{360}{n}$

For (n - 1) sided regular polygon = $\frac{360}{n - 1}$

For (n + 2) sided regular polygon = $\frac{360}{n + 1}$

⇒ $\frac{360}{n - 1} - \frac{360}{n + 2}$ = 6 9Given)

⇒ $\frac{360(n + 2) - 360(n - 1)}{(n - 1)(n + 2)}$

⇒ $\frac{360n + 720 - 360n + 360}{(n - 1)(n + 2)}$

⇒ $\frac{1080}{(n - 1)(n + 2)} = \frac{6}{1}$

⇒ 1080 = 6 (n - 1)(n + 2)

⇒ 180 = (n - 1)(n + 2)

⇒ 180 = n$^2$+ 2n - n - 2

⇒ 180 = n$^2$ + n - 2

⇒ n$^2$b+ n - 2 - 180 = 0

⇒ n$^2$ + n - 182 = 0

⇒ n$^2$ + 14n - 13n - 182 = 0

⇒ n (n + 14) - 13 (n + 14) = 0

⇒ (n - 13) (n + 14) = 0

⇒ n - 13 = 0 or n + 14 = 0

⇒ n = 13 or n = -14

∴ n = 13 (We can't have a negative number of side)